Section 6.2 Voting Methods
Objectives: Section 6.2
Students will be able to:
Read a voter preference schedule for ranked choice voting
Calculate the minimum number of votes to win a majority
Find the winner of an election using the plurality method
Find the winner of an election using the instant runoff method
Find the winner of an election using the Borda count method
Find the winner of an election using the pairwise (Condorcet) method
Subsection 6.2.1 Voting Rights
Now that we have studied how to apportion the number of representatives for each state, along with a brief history of the U.S. government, we want to look at how representatives and other public officials are elected. There are also many other situations where a group consensus is needed, and some type of voting occurs. You may be most familiar with systems where you get one vote and the candidate with the most votes wins. However, there are many other ways of determining a winner. We will look at several voting methods in this section.
For an election using any of these methods to be fair, each person must have an equal right to vote and an equal ability to exercise that right. The Voting Rights Act of 1965 prohibited any election practice that denies the right to vote based on race, but key portions of the law have been invalidated since then. Voter suppression continues today in different forms such as purging voter rolls, eliminating polling places and voter identification laws, which can also affect transgender people.
The John Lewis Voting Rights Advancement Act was passed in the U.S. House of Representatives in 2019 to restore elements of the Voting Rights Act and make it possible to enforce the law. It has not been taken up in the Senate, though. Follow this link if you would like to read a more detailed timeline of voting rights 71 .
Subsection 6.2.2 Ranked Choice Ballots
To use some of the methods we are going to study, we need to know more than just each person’s first choice. We also need to know their 2nd and 3rd choices, and so on. A ballot where each person ranks all of the choices in order of their preference is called a ranked choice ballot.
The image to the right shows an example of a ranked choice ballot 72 . Ranked choice ballots can be used in elections by mail or in person at polling places. Let’s see how to tally the results from a ranked choice election.
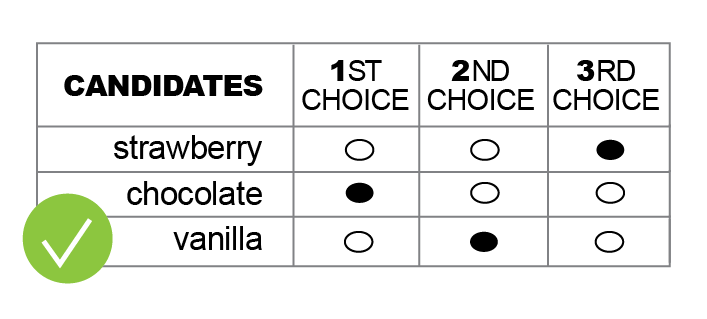
Example 6.2.2.
Students are voting for their class president and the candidates are Omar (O), Ana (A), and Helena (H). They have ranked the candidates according to their preference.
| Vien | Ana | Marv | Tasha | Eve | Omar | Lupe | Dave | Helena | Jimmy | |
|---|---|---|---|---|---|---|---|---|---|---|
| 1st choice | A | A | O | H | A | O | H | O | H | A |
| 2nd choice | O | H | H | A | H | H | A | H | A | H |
| 3rd choice | H | O | A | O | O | A | O | A | O | O |
Subsection 6.2.3 Preference Schedule
To simplify this data, we create a preference schedule which combines all the people who voted with the exact same ranking. For example, Tasha, Lupe, and Helena, all voted in the same way, HAO. So, we will list that combination once, with the number 3 at the top of that column. Three students also voted for AHO, and OHA. One student voted for the ranking AOH. Below is the preference schedule.
| Number of voters | 1 | 3 | 3 | 3 |
| 1st choice | A | A | O | H |
| 2nd choice | O | H | H | A |
| 3rd choice | H | O | A | O |
Notice that by totaling the vote counts across the top of the preference schedule we can see the total number of votes cast: \(1+3+3+3 = 10\) total votes.
Subsection 6.2.4 Plurality Method
The voting method you may be most familiar with in the United States is the plurality method. In the plurality method, the candidate with the most first-choice votes is declared the winner.
There is a difference between a majority and a plurality. For a majority, a candidate must have over 50% of the votes. There are some states that require a majority in order to win an election. In more cases, though, only a plurality is required, which means having more votes than any other candidate. If a tie occurs without using ranked choice voting, then a new run-off election would be required.
Example 6.2.3.
In our election from above, we had this preference table:
| Number of voters | 1 | 3 | 3 | 3 |
| 1st choice | A | A | O | H |
| 2nd choice | O | H | H | A |
| 3rd choice | H | O | A | O |
For the plurality method, we only look at the 1st choice row. Totaling them up:
Ana: \(1+3 = 4\) votes
Omar: \(3\) votes
Helena: \(3\) votes
Ana is the winner using the plurality voting method.
Notice that Ana won with 4 out of 10 votes, or 40% of the votes, which is a plurality of the votes, but not a majority.
Subsection 6.2.5 How Many Votes are Needed to Win?
If we know how many voters there are, we can calculate the minimum number of votes needed for a majority win.
Continuing Example 6.2.3 from above, there are 10 voters, so we divide that by 2 or multiply by 0.5 to find 50%. Then we round up to the next whole number to get just over 50%.
\(10 \div 2=5\text{.}\) Then we round up to 6 votes.
Exactly half of the votes would be a tie, so we round up to the next whole number. A candidate would need 6 out of 10 votes to win a majority. If there was an odd number of voters we would divide by 2 and get a decimal. Let’s say there were 13 voters:
\(13 \div 2=6.5\text{.}\) Then we round up to 7 votes.
Then we round up to the next whole number, which is 7 votes. A candidate can win a plurality with fewer votes than the majority, as long as they have more than any other candidate.
Subsection 6.2.6 Insincere Voting
In a system with only two major political parties like the United States, it can be nearly impossible for third-party candidates to break through. You may feel like you would be wasting your vote if you voted for a third-party candidate. Consider this example.
Example 6.2.4.
Here is a two-party election with preferences shown below. It is a close race, but candidate B would win.
| Number of voters | 96 | 100 |
| 1st choice | A | B |
| 2nd choice | B | A |
Suppose a third candidate, C, entered the race, and a segment of voters sincerely want to vote for that third candidate. Here is the new preference schedule.
| Number of voters | 96 | 90 | 10 |
| 1st choice | A | B | C |
| 2nd choice | B | A | B |
| 3rd choice | C | C | A |
The ten people who prefer candidate C are in a bind because if they vote for C, then A will win, but they prefer B over A. It is likely that they will vote for B, their second choice, to keep A from winning.
Situations like this can lead to insincere voting. Insincere voting is when a person doesn’t vote according to their actual preference for strategic purposes. In the case above, a person who wants to vote for a third-party candidate realizes it would take a vote away from their major party candidate. Not wanting to see their party lose the election, they vote for the major party candidate.
Subsection 6.2.7 Instant Runoff Method
The Instant Runoff Method is a modification of the plurality method that attempts to address the issue of insincere voting.
The voting is done with ranked choice ballots and a preference schedule is generated. Then we tally all the first-choice votes. The candidate with the least first-place votes is eliminated, but any votes for that candidate are transferred to the voters’ next choice. The process continues until one candidate has a majority. We will show you how it works with an example.
Example 6.2.5.
Consider the preference schedule below, in which a committee is choosing a chairperson. The candidates are labeled A, B, C, D, and E for simplicity. Here is the preference schedule:
| Number of voters | 3 | 5 | 1 | 5 | 7 | 2 | 1 |
| 1st choice | B | C | C | B | D | A | E |
| 2nd choice | C | A | B | D | C | E | D |
| 3rd choice | A | E | B | C | A | B | B |
| 4th choice | D | D | E | A | E | C | A |
| 5th choice | E | B | A | E | B | D | C |
Let’s start by tallying the first-choices.
A: \(2\)
B: \(3+5=8\)
C: \(5+1=6\)
D: \(7\)
E: \(1\)
If this was a plurality election, B would be the winner with 8 first-choice votes.
Round 1.
We make our first elimination. Choice E has the fewest first-place votes, so we eliminate E from the election. To transfer the 1 vote, we look at the person who voted for E in the last column. Their second choice was D, so we transfer that one vote to D, who will now have 8 votes:
A: \(2\)
B: \(8\)
C: \(6\)
D: \(7+1 = 8\)
E: \(1\)
Round 2.
There are 24 votes in total, so a majority would be 13 votes. Since no candidate has the majority, we eliminate the next candidate with the fewest votes, which is A. We look at the column for the two people who chose A. Their second choice was E, who is already eliminated. Their third choice is B, so their 2 votes are transferred to B, for a total of 10:
A: \(2\)
B: \(8+2=10\)
C: \(6\)
D: \(8\)
E: \(1\)
Round 3.
Now C has the fewest votes, and there are two columns for C in the preference schedule. Five people who voted for C chose A second, then E (who have already been eliminated), then D. So, we transfer their 5 votes to D. One person who voted for C selected B as their second choice, so their one vote goes to B. Then we tally the votes for each remaining candidate.
A: \(2\)
B: \(10+1=11\)
C: \(6\)
D: \(8+5=13\)
E: \(1\)
Now we can see that D is the winner of the instant runoff method with 13 votes. We can stop this method earlier if a candidate reaches a majority.
The Instant Runoff Method is similar to the idea of holding runoff elections, but since every voter’s order of preference is recorded on the ballot, the runoff can be computed quickly without requiring a second costly election. It also makes it safe to vote for a third-party candidate knowing that your vote will go to your second choice if your first choice can’t win.
This voting method is used in several places around the world, including the election of members of the Australian House of Representatives, statewide elections in Maine, and local elections in Benton County, Oregon (Ranked Choice Voting Resource Center, 2020).
Subsection 6.2.8 Borda Count (Point System)
Borda Count is another voting method, named for Jean-Charles de Borda, who developed the system in 1770. In this method, points are assigned to each candidate based on their ranking; 1 point for last choice, 2 points for second-to-last choice, and so on. The point values are totaled, and the candidate with the largest point total is the winner. We’ll show you how to do it in the next example.
Example 6.2.6.
A group of PCC students are getting together for a student leadership conference and they need to decide where to meet. Their members are from four campuses: Sylvania, Rock Creek, Cascade and Southeast. The votes for where to hold the conference were:
| Number of voters | 31 | 25 | 10 | 14 |
| 1st choice | Sylvania | Rock Creek | Southeast | Cascade |
| 2nd choice | Southeast | Cascade | Cascade | Rock Creek |
| 3rd choice | Rock Creek | Sylvania | Sylvania | Southeast |
| 4th choice | Cascade | Southeast | Rock Creek | Sylvania |
Now we will add a column on the left side of the table for the points. Then we multiply the points by the number of voters who put each candidate in that rank,
| Points | Number of voters | 31 | 25 | 10 | 14 |
| 4 | 1st choice | Sylvania | Rock Creek | Southeast | Cascade |
| 3 | 2nd choice | Southeast | Cascade | Cascade | Rock Creek |
| 2 | 3rd choice | Rock Creek | Sylvania | Sylvania | Southeast |
| 1 | 4th choice | Cascade | Southeast | Rock Creek | Sylvania |
We will start with Sylvania in the bottom row and move up. They have one point times 14 voters, 2 points times 25 + 10 voters, 3 points times zero, and 4 points times 31 voters. Multiplying and then adding gives us a total of 208 points for Sylvania. You can see the calculation below and we will do the same thing for each campus:
Sylvania: \(1 \cdot 14 + 2 \cdot 35 + 3 \cdot 0 + 4 \cdot 31=208 \) points
Rock Creek: \(1 \cdot 10 + 2 \cdot 31 + 3 \cdot 14 + 4 \cdot 25= 214\) points
Cascade: \(1 \cdot 31 + 2 \cdot 0 + 3 \cdot 35 + 4 \cdot 14= 192\) points
Southwest: \(1 \cdot 25 + 2 \cdot 14 + 3 \cdot 31 + 4 \cdot 10 = 186\) points
Using the Borda count method, Rock Creek is the winning location.
Note that Sylvania would have won with the plurality method. Borda count is sometimes described as a consensus-based voting system, since it can be used to choose a more broadly acceptable option over the one with the most first-choice support. This is a different approach than plurality and instant runoff voting that focus on first-choice votes; Borda Count considers every voter’s entire ranking to determine the outcome.
Because of this consensus behavior, Borda Count, or some variation of it, is commonly used in awarding sports awards. Variations are used to determine the Most Valuable Player in baseball, to rank teams in NCAA sports, and to award the Heisman trophy.
Subsection 6.2.9 Pairwise Comparison: Copeland’s Method
Another method, called pairwise comparison, the Condorcet method or Copeland’s method, attempts to be fair by looking at each pair of candidates separately.
In this method, we look at each pair as if they were the only two candidates running and determine which of the two is more preferred. In Copeland’s method, the more preferred candidate is awarded 1 point. If there is a tie, each candidate is awarded \(\frac{1}{2}\) point. After all the pairwise comparisons are made, the candidate with the most points, and hence the most pairwise wins, is declared the winner.
Variations of Copeland’s Method are used in many professional organizations, including election of the Board of Trustees for the Wikimedia Foundation that runs Wikipedia.
Example 6.2.7.
Consider our class president example from the beginning of the chapter. Determine the winner using Copeland’s Method.
| Number of voters | 1 | 3 | 3 | 3 |
| 1st choice | A | A | O | H |
| 2nd choice | O | H | H | A |
| 3rd choice | H | O | A | O |
We need to look at each pair of candidates to see which would win in a one-to-one comparison. First, we will systematically list all of the pairs of candidates:
Helena vs. Omar
Helena vs. Ana
Ana vs. Omar
Next, comparing Helena with Omar, we see that 6 voters, those marked in bold in the table below, would prefer Helena over Omar. Note that Helena doesn’t have to be the voter’s first choice – we’re imagining that Ana wasn’t in the race. The other 4 people chose Omar over Helena.
| Number of voters | 1 | 3 | 3 | 3 |
| 1st choice | A | A | O | H |
| 2nd choice | O | H | H | A |
| 3rd choice | H | O | A | O |
Based on this comparison of Helena vs. Omar, Helena wins 1 point.
Next, comparing Helena with Ana, the 6 voters in the last two columns prefer Helena to Ana, so Helena gets one point. Lastly, we compare Ana with Omar. The 1 voter in the first column prefers Ana, as do the 3 voters in the second column. The 3 voters in the third column prefer Omar, but the 3 voters in the last column would choose Ana. So, altogether \(1+3+3=7\) voters prefer Ana over Omar. Ana gets 1 point.
To summarize, we write how many voters prefer each candidate ignoring all other candidates:
Helena-6 vs Omar-4 (Helena gets 1 point)
Helena-6 vs Ana-4 (Helena gets 1 point)
Ana-7 vs Omar-3 (Ana gets 1 point)
Ana has a total of 1 point and Helena has 2 points, so Helena is the winner under Copeland’s Method. This method gave us a different winner than the plurality method where Ana won, because more people preferred Helena to Ana, even though they didn’t choose Helena for their first choice.
Now that we’ve seen an example of each voting method, let’s run through each method for the same scenario.
Example 6.2.8.
Consider an advertising team voting to choose one of four different slogans, labeled A, B, C and D. Determine the winner using each method we have learned.
| Number of voters | 5 | 3 | 6 | 4 | 2 |
| 1st choice | D | A | C | B | A |
| 2nd choice | A | C | B | D | D |
| 3rd choice | C | B | A | A | C |
| 4th choice | B | D | D | C | B |
Plurality Method: We tally the first-place votes:
A: \(3+2=5\)
B: \(4\)
C: \(6\)
D: \(5\)
C wins the plurality method.
Instant Runoff Method: We start with the plurality tallies. Then we look for the slogan with the least first-choice votes, and that is B. Looking in the preference schedule at the 4 people who voted for B, we see their second choice is D. So we transfer those 4 votes to D, which now has 9.
A: \(5\)
B: \(4\)
C: \(6\)
D: \(5+4=9\)
Now A has the least number of votes with 5, so we look at the voters who chose A for their first choice. There are two columns, so three of those votes will be transferred to C and 2 will be transferred to D.
A: \(5\)
B: \(4\)
C: \(6+3=9\)
D: \(9+2=11\)
D is the winner of the instant runoff method.
Borda Count: We add a column on the left for the points, starting with 1 point for last place and counting up. Then we multiply the points by the number of votes and add them all up.
| Points | Number of voters | 5 | 3 | 6 | 4 | 2 |
| 4 | 1st choice | D | A | C | B | A |
| 3 | 2nd choice | A | C | B | D | D |
| 2 | 3rd choice | C | B | A | A | C |
| 1 | 4th choice | B | D | D | C | B |
A: \(1 \cdot 0 + 2 \cdot 10 + 3 \cdot 5 + 4 \cdot 5 = 55\) points
B: \(1 \cdot 7 + 2 \cdot 3 + 3 \cdot 6 + 4 \cdot 4 = 47\) points
C: \(1 \cdot 4 + 2 \cdot 7 + 3 \cdot 3 + 4 \cdot 6 = 51\) points
D: \(1 \cdot 9 + 2 \cdot 0 + 3 \cdot 6 + 4 \cdot 5 = 47\) points
A is the winner of the Borda count method.
Pairwise Comparison:
First we list all the possible pairs:
A vs B
A vs C
A vs D
B vs C
B vs D
C vs D
Then we count the number of voters who would choose each candidate if they were the only two in the race.
A-10 vs B-10
A-14 vs C-6
A-11 vs D-9
B–4 vs C-16
B-13 vs D-7
C-9 vs D-11
Totaling one point for each win and half a point for each tie gives us:
A has 2.5 points
B has 1.5 points
C has 1 point
D has 1 point
A is the winner of the pairwise comparison method.
Subsection 6.2.10 Which Method is the Most Fair?
To see a very simple example of how difficult voting can be, consider the election below:
| Number of voters | 5 | 5 | 5 |
| 1st choice | A | C | B |
| 2nd choice | B | A | C |
| 3rd choice | C | B | A |
Notice that in this election: 10 people prefer A to B, 10 people prefer B to C, and 10 people prefer C to A.
No matter whom we choose as the winner, \(\frac{2}{3}\) of voters would prefer someone else! This scenario is called Condorcet’s Voting Paradox. In this situation, there is no fair resolution. There are many additional paradoxes and fairness criteria that are explained in David Lippman’s original version of Math in Society 76 .
Since it is not possible to have one method that is fair in every situation, it is important to decide which method is the most fair for any given situation.
Subsection 6.2.11 Primaries and Sequential Voting
For many public offices in the U.S., a sequence of two public votes is held: a primary election and the general election. For non-partisan offices like sheriff and judge, in which political party affiliation is not declared, the primary election is usually used to narrow the field of candidates.
For partisan positions, typically, either the top two candidates receiving the most votes move forward, or the top candidate from each party will move forward. This is called sequential voting - a process in which voters cast totally new ballots after each round of eliminations. Sequential voting has become quite common in television, where it is used in reality shows like The Voice.
In some states a closed primary is used, in which only voters of each party can vote for that party’s candidates. In other states, an open primary is used, where voters can pick the party whose primary they want to vote in. In other states, caucuses are used, which are meetings of the political parties, only open to party members. Closed primaries are often disliked by independent voters, who like the flexibility to change which party they are voting in. Open primaries have the disadvantage of raiding, though, where a voter could vote in their non-preferred party’s primary with the intent of selecting a weaker opponent for their preferred party’s candidate.
Regardless of the primary type, the general election is the main election. While rules vary state-to-state, for an independent or minor party candidate to get listed on the ballot, they typically have to gather a certain number of signatures to petition for inclusion.
In the next section we will look at a different type of election for the President of the United States.
Exercises 6.2.12 Exercises
1.
To decide on a new website design, the designer asks people to rank three designs that have been created (labeled A, B, and C). The individual ballots are shown below. Create a preference table.
ABC, ABC, ACB, BAC, BCA, BCA, ACB, CAB, CAB, BCA, ACB, ABC
2.
To decide on a movie to watch, a group of friends all vote for one of the choices (labeled A, B, and C). The individual ballots are shown below. Create a preference table.
CAB, CBA, BAC, BCA, CBA, ABC, ABC, CBA, BCA, CAB, CAB, BAC
Exercise Group.
In exercises 3-18, complete the following:
How many voters voted in this election?
How many votes are needed for a majority?
Find the winner under the plurality method.
Find the winner under the Instant Runoff Voting method.
Find the winner under the Borda Count Method.
Find the winner under Copeland’s method.
3.
The planning committee for a renewable energy trade show is trying to decide where to hold their next trade show in. The votes are shown below.
| Number of voters | 9 | 19 | 11 | 8 |
| 1st choice | Buffalo | Atlanta | Chicago | Buffalo |
| 2nd choice | Atlanta | Buffalo | Buffalo | Chicago |
| 3rd choice | Chigaco | Chicago | Atlanta | Atlanta |
4.
A non-profit agency is electing a new chair of the board. The votes are shown below.
| Number of voters | 11 | 5 | 10 | 4 | 3 |
| 1st choice | Abdulla | Cantos | Beck | Cantos | Abdulla |
| 2nd choice | Cantos | Beck | Cantos | Abdulla | Beck |
| 3rd choice | Beck | Abdulla | Abdulla | Beck | Cantos |
5.
A group of students is deciding which class to take together. The votes are shown below.
| Number of voters | 2 | 1 | 2 | 3 | 2 | 2 |
| 1st choice | Art | Art | Biology | Biology | Calculus | Calculus |
| 2nd choice | Biology | Calculus | Art | Calculus | Art | Biology |
| 3rd choice | Calculus | Biology | Calculus | Art | Biology | Art |
6.
A professional psychology association is deciding where to hold their next conference. The votes are shown below.
| Number of voters | 3 | 2 | 7 | 3 | 6 | 5 |
| 1st choice | Alaska | Alaska | Barbados | Barbados | California | California |
| 2nd choice | Barbados | California | Alaska | California | Alaska | Barbados |
| 3rd choice | California | Barbados | California | Alaska | Barbados | Alaska |
7.
The Hillsboro City Council has an election with three candidates, D, E, F. The votes are:
| Number of voters | 5 | 4 | 3 | 7 | 2 | 10 |
| 1st choice | D | D | E | E | F | F |
| 2nd choice | E | F | F | D | E | D |
| 3rd choice | F | E | D | F | D | E |
8.
The Vancouver mayor’s race has three candidates, G, H, I. The votes are:
| Number of voters | 12 | 6 | 14 | 3 | 15 | 1 |
| ast choice | G | G | H | H | I | I |
| 2nd choice | H | I | I | G | G | H |
| 3rd choice | I | H | G | I | H | G |
9.
The Beaverton City Council has an election for one open position. There are four candidates (labeled A, B, C, and D for convenience). The preference schedule for the election is:
| Number of voters | 120 | 50 | 40 | 90 | 60 | 100 |
| 1st choice | C | B | D | A | A | D |
| 2nd choice | D | C | A | C | D | B |
| 3rd choice | B | A | B | B | C | A |
| 4th choice | A | D | C | D | B | C |
10.
Portland City Council has an election for one open position. There are four candidates (labeled E, F, G, H for convenience). The preference schedule for the election is:
| Number of voters | 11 | 8 | 3 | 9 | 16 | 14 | 9 |
| 1st choice | E | H | H | G | E | F | F |
| 2nd choice | F | G | E | F | G | H | G |
| 3rd choice | H | F | G | H | H | E | E |
| 4th choice | G | E | F | E | F | G | H |
11.
The Portland mayor’s race has four candidates, I, J, K, L. The votes are:
| Number of voters | 21 | 18 | 9 | 14 | 5 | 12 | 11 | 2 |
| 1st choice | I | K | K | L | J | I | K | J |
| 2nd choice | L | I | J | K | I | K | L | I |
| 3rd choice | J | L | I | I | K | L | I | L |
| 4th choice | K | J | L | J | L | J | J | K |
12.
A Reynolds School Board position has four candidates, M, N, O, P. The votes are:
| Number of voters | 16 | 2 | 12 | 15 | 34 | 22 | 32 | 13 | 11 |
| 1st choice | M | N | N | P | O | M | M | O | N |
| 2nd choice | O | O | P | O | M | P | N | M | O |
| 3rd choice | P | M | O | N | N | O | P | P | M |
| 4th choice | N | P | M | M | P | N | O | N | P |
13.
The Gresham mayor’s race has four candidates, Q, R, S, T. The votes are:
| Number of voters | 11 | 4 | 15 | 18 | 14 | 5 | 13 | 10 |
| 1st choice | Q | S | Q | T | R | S | S | R |
| 2nd choice | R | T | S | S | Q | Q | Q | T |
| 3rd choice | S | R | T | Q | T | T | R | S |
| 4th choice | T | Q | R | R | S | R | T | Q |
14.
The Parkrose School Board has an election for an open position with four candidates, U, V, W, X. The votes are:
| Number of voters | 16 | 21 | 15 | 5 | 3 | 11 | 9 | 8 | 22 |
| 1st choice | U | W | X | V | X | X | W | U | V |
| 2nd choice | X | X | V | X | W | U | V | V | W |
| 3rd choice | V | U | U | W | V | V | X | X | U |
| 4th choice | W | V | W | U | U | W | U | W | X |
15.
A Multnomah county commissioner’s race has five candidates, A, B, C, D, E. The votes are:
| Number of voters | 12 | 14 | 21 | 18 | 19 | 23 |
| 1st choice | B | C | E | E | A | B |
| 2nd choice | A | A | B | A | C | D |
| 3rd choice | D | E | A | B | B | C |
| 4th choice | E | B | C | C | D | E |
| 5th choice | C | D | D | D | E | A |
16.
The Oregon State governor’s race has five candidates, F, G, H, I, J. The votes are:
| Number of voters | 16 | 12 | 9 | 21 | 17 | 11 | 6 |
| 1st choice | F | F | H | I | G | G | J |
| 2nd choice | I | G | I | F | H | J | F |
| 3rd choice | G | H | G | J | I | F | H |
| 4th choice | J | J | J | H | F | I | G |
| 5th choice | H | I | F | G | J | H | I |
17.
A Washington county commissioner’s race has five candidates, K, L, M, N, O. The votes are:
| Number of voters | 22 | 19 | 13 | 26 | 14 | 5 | 17 | 11 |
| 1st choice | K | M | K | O | L | L | N | N |
| 2nd choice | M | L | L | K | N | K | O | L |
| 3rd choice | L | N | N | N | M | O | K | K |
| 4th choice | N | O | M | L | K | M | M | O |
| 5th choice | O | K | O | M | O | N | L | N |
18.
A Clackamas county commissioner’s race has five candidates, P, Q, R, S, T. The votes are:
| Number of voters | 31 | 16 | 29 | 52 | 16 | 18 | 11 | 13 | 12 |
| 1st choice | R | R | P | Q | T | T | S | S | S |
| 2nd choice | S | Q | R | S | Q | S | T | Q | P |
| 3rd choice | T | T | T | R | S | Q | R | T | R |
| 4th choice | P | S | Q | T | P | R | P | R | T |
| 5th choice | Q | P | S | P | R | P | Q | P | Q |
https://www.rankedchoicevoting.org/mark_rcv_ballothttps://www.rankedchoicevoting.org/http://creativecommons.org/licenses/by-sa/3.0http://www.opentextbookstore.com/mathinsociety/index.html